Kirchhoff’s Laws And Their Application
Kirchhoff extended Ohm’s law to networks, and gave two laws, which enable current in any part of the network to be calculated.
Kirchhoff’s first law
In a circuit, current may divide up where circuit splits into two separate branches. This doesn’t mean that current will disappear or an extra current will show up from nowhere. This is the basis for Kirchhoff’s first law.
Kirchhoff’s first law states that the current entering any point in a circuit is equal to the sum of the currents leaving the same point. Said differently, the algebraic sum of the currents into any junction is zero.
This law is also called Kirchhoff’s current law. It is an expression of conservation of charge i.e. the amount of charge entering a point must leave the same point. Kirchhoff’s first law obeys principle of conservation of charge.

Kirchhoff’s second law
This law deals with electromotive force (e.m.f.s) and the voltages in a circuit.
Kirchhoff’s second law states that the sum of e.m.f.s around any loop in a circuit is equal to the sum of the p.d.s (potential difference) around the loop.

Kirchhoff’s second law is a consequence of the principle of conservation of energy i.e. energy gain passing through sources of e.m.f = energy lost passing through components with p.d.s. Kirchhoff’s second law obeys principle of conservation of energy.
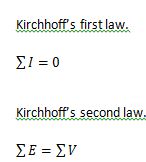
Kirchhoff’s Applications (Examples)
Ex 1
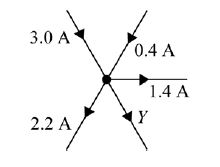
The diagram shows a junction in a circuit. What is the current Y ?
A +0.2 A
B −0.2 A
C +1.8 A
D −1.8 A
Solution
Current flowing to th point = Current leaving the point
3 + 0.4 = 2.2 + Y + 1.4
3.4 = 3.6 + Y
Y = 3.4 – 3.6 =- 0.2 A
Ex 2
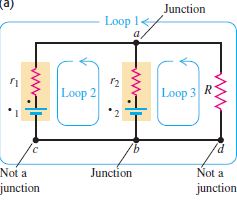
Use Kirchhoff’s laws to determine the currents I1, I2 and I3 in the circuit on the right.

Solution
I2 + I3 = I1 (obeying Kirchhoff’s first law)
Using Kirchhoff’s second law to solve the loop
Ist loop (Clockwise direction)
3 = 10 * I1 +20*I2 (eq 1)
2nd loop (anti-clockwise direction)
1.5 = 20* I2 (eq 2)
I2 = 1.5/20 = 0.075 A
subtituting I2 in eq 1
3 = 10*I1 + 1.5
1.5 = 10* I1
I1 = 0.15 A
I2 + I3 = I1
0.075 + I3 = 0.15
I3 = 0.15 – 0.075 = 0.075 A
Recommended: Note on Direct Current
