In this article, I will show the kinematic equations for both linear and angular, how they are derived, and graphs. Kinematics in physics is about the motion of a body or an object. And the terms associated with kinematics are displacement, speed, velocity, and acceleration. These are the variables physicists play around in this topic.
Kinematics equations are a set of equations that can derive an unknown aspect of a body’s motion if the other aspects are provided.
The starting point is from the definition of acceleration which is the rate of change of velocity. This is like a car traveling at a certain speed then the driver decides to move faster or slower by pressing the accelerator. The speedometer in the car will change and at that instance, the car has to either accelerate or decelerate.
First Equation
Acceleration = change of velocity / time = (v – u) / t
u is the initial velocity, v is the final velocity, t is the time taken, and a is the acceleration
cross multiply,
Make v the subject of the equation
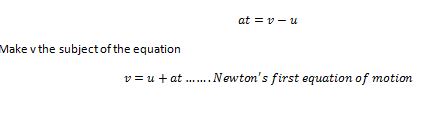
The first equation above is the Newton’s first equation of motion
Second equation
The next step,
From average velocity,
Average velocity = s / t
Average velocity = (v +u)/2
Equate the two equations together, you get
s / t = (v +u)/2
Cross multiply and rearrange
s = [(v + u)t]/2
substitute v = u + at into s = [(v + u)t]/2
you have
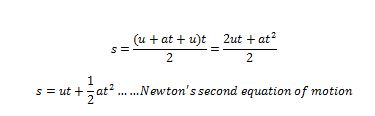
This is the Newton’s second equation of motion
Recommended: Short notes on motion
Third equation
Final step:
Make t the subject of the Newton’s first equation of motion
t = (v – u) / a
substitute t = (v – u) / a into Newton’s second equation of motion
Cross multiply,
This is the Newton’s third equation of motion
Angular Kinematics Equations
The equation above is the linear kinematic equations but here we will look at the branch which deals with the rotational motion of anybody. In this case,
- Displacement is replaced by a change in angle.
- Initial and final velocities are replaced by initial and final angular velocities.
- Acceleration is replaced by angular
- Time is the only constant.
Kinematic Graphs
The next thing is to look at Kinematics graphs. The graphs are related to the equations that I have given above. The velocity graph is the derivative of the displacement graph while the acceleration-time graph is the derivative of a velocity-time graph in kinematics.
